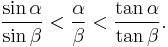Aristarchus' inequality

In trigonometry, Aristarchus' inequality, named after the ancient astronomer Aristarchus of Samos, states that if α and β are acute angles (i.e. between 0 and a right angle) and β < α then

\frac{\sin\alpha}{\sin\beta} < \frac{\alpha}{\beta} < \frac{\tan\alpha}{\tan\beta}.

The first of these inequalities was used by Ptolemy in constructing his table of chords.[1]

Notes and references

  1. ^ Toomer, G. J. (1998), Ptolemy's Almagest, Princeton University Press, ISBN 0-691-00260-6 

External links